More Information
Submitted: 05 February 2020 | Approved: 18 February 2020 | Published: 19 February 2020
How to cite this article: Ferro P. Raw materials criticalities in material selection & design. Int J Phys Res Appl. 2020; 3: 017-019.
DOI: 10.29328/journal.ijpra.1001020
ORCiD: orcid.org/0000-0001-8008-1557
Copyright License: © 2020 Ferro P. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Raw materials criticalities in material selection & design
Paolo Ferro*
Department of Engineering and Management, University of Padova, Vicenza, Italy
*Address for Correspondence: Paolo Ferro, Department of Engineering and Management, University of Padova, Stradella S. Nicola 3, 36100 Vicenza, Italy, Tel: +39 344 6957226; Email: paolo.ferro@unipd.it
Circular Economy, Sustainability, Design for Environment are some of the keywords that identify new formidable challenges to be faced in the next years. Raw materials have a dominant role in reaching that goal. Green energy, electric vehicles, communication, etc. depends on raw materials labeled as critical because of their economic importance coupled with high supply risk. For this reason, mitigating actions need to be used in materials selection and design such as material substitution, improved materials efficiency and recycling. In this technical communication, a method to implement raw materials criticality issues in materials selection is described according to the recent literature. The strategy is based on Ashby’s approach and the definition of the alloy criticality index quantifying the criticality per unit of mass of the material.
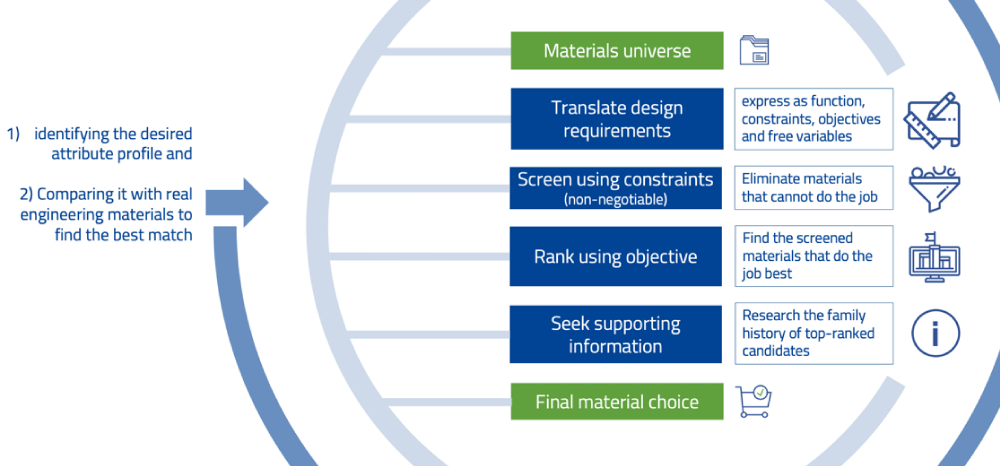
Materials selection should accompany all the phases of the design process, from the concept to the details. The consequences of choices made at the concept or embodiment stages may not become apparent until the detail is examined. Iteration, looping back to explore alternatives, is an essential part of the design process. Thus, the materials selection strategy must be systematic and easy to apply. In 2004, Ashby, et al. [1] published a paper dealing with a powerful method to select materials and processes. It consists of four main steps (Figure 1). Starting from the materials universe, design requirements have to be first translated in terms of constraints, free variables and objectives to optimize. All materials are then screened according to constraints and the ‘surviving materials’ are ranked using the objective. Finally, supporting information is required to select the best material. The method requires a database in which physical, chemical, thermo-mechanical properties are stored for each material.
Figure 1: Materials selection procedure by Ashby, et al. [1].
An interesting concept of the Ashby’s method is the definition of the material index that is used to rank the surviving materials. Starting from the objective equation, it is calculated by eliminating the free variable through the constraint equation. For example, if the material that minimizes the mass (m) of a tie rod is to be select, the objective equation is:
Where ρ is the material density, L is the length and A is the cross section (free variable) of the component. If the tie rod stiffness (S) is the constraint to take into account,
With E = Young’s modulus, the free variable is obtained from Eq. (2) and substituted into Eq. (1) obtaining:
With fixed values of L and S, the lower the ratio ρ/E, the lower the mass of the tie rod. ρ/E is called material index and it is a function of material proprieties only. Commonly, it is used its inverse expression (say, M = E/ρ) with the aim to optimize the objective equation (Eq. (3)) by maximizing the index M. Now the question is: which is the objective equation used to select materials in a critical raw materials (CRMs) perspective? To answer this question, it is necessary first to quantify the criticality issues of a generic raw material.
Criticalities assessment
Criticality issues linked to each raw material are quantified by a series of indexes such as the abundance risk (ARL), the sourcing and geopolitical risk (SGR), the environmental country risk (ECR), the normalized supply risk (NSR), the economic importance (EI) and finally, the recycling drawback index (RDI) [3]. In order to use such indicators in design, it is necessary to aggregate them in an overall general indicator for each critical raw material (i) (CICRMi):
In Eq. (4) k is a non-dimensional coefficient which value is in between 0 and 1, according to the seriousness of the corresponding criticality aspect. When all k values are set equal to 1 in Eq. (4), equal seriousness is perceived for all the criticality aspects. The values of the criticality index in Eq. (4) are calculated by using data taken from the literature [3]. Table 1 collects the numerical values of each criticality index. It is observed that the high seriousness of the European Union dependence from rare earths is reflected by the highest values of their criticality indicator.
| Table 1: Raw materials criticality indexes elaborated starting from values coming from the European Commission evaluations [2]. *LREEs: Light Rare Earth Elements; **HREEs: Heavy Rare Earth Elements | |||||||
| CRM | ARL | SGR | ECR | NSR | NEI | RDI | CICRM |
| Sb | 6.15 | 6.46 | 7.68 | 8.78 | 5.89 | 3.64 | 6.43 |
| Ba | 2.82 | 2.59 | 2.62 | 3.27 | 3.97 | 9.77 | 4.17 |
| Be | 5.00 | 4.49 | 6.43 | 4.90 | 5.34 | 10.00 | 6.03 |
| Bi | 7.52 | 7.18 | 8.52 | 7.76 | 4.93 | 9.77 | 7.61 |
| B | 4.45 | 5.04 | 5.31 | 6.12 | 4.25 | 10.00 | 5.86 |
| Ce (LREEs*) | 3.63 | 10.00 | 9.49 | 10.00 | 4.93 | 9.77 | 7.97 |
| Co | 4.05 | 4.20 | 3.94 | 3.27 | 7.81 | 10.00 | 5.55 |
| F | 2.68 | - | - | 2.65 | 5.75 | 9.77 | - |
| Ga | 4.17 | 6.88 | 8.19 | 2.86 | 4.38 | 10.00 | 6.08 |
| Ge | 5.27 | 6.97 | 8.33 | 3.88 | 4.79 | 9.55 | 6.46 |
| Hf | 4.97 | 1.31 | 2.02 | 2.65 | 5.75 | 9.77 | 4.41 |
| He | - | - | - | 3.27 | 3.56 | 9.77 | - |
| In | 6.05 | 3.57 | 3.97 | 4.90 | 4.25 | 10.00 | 5.46 |
| Ir | 8.45 | 5.49 | 6.66 | 5.71 | 5.89 | 6.82 | 6.50 |
| La (LREEs*) | 3.86 | 8.40 | 10.00 | 10.00 | 4.93 | 9.77 | 7.83 |
| Mg | 1.08 | 7.85 | 9.33 | 8.16 | 9.73 | 7.95 | 7.35 |
| Natural graphite (carbon) | 3.15 | 6.98 | 8.33 | 5.92 | 3.97 | 9.32 | 6.28 |
| Nb | 4.15 | 5.48 | 6.17 | 6.33 | 6.58 | 9.93 | 6.44 |
| Pd | 7.27 | 3.11 | 3.11 | 3.47 | 7.67 | 7.73 | 5.39 |
| P | 2.43 | - | - | 2.04 | 6.99 | 6.14 | - |
| Pt | 7.75 | 3.93 | 4.71 | 4.49 | 6.71 | 7.50 | 5.85 |
| Pr | 4.49 | 8.40 | 10.00 | 10.00 | 4.93 | 7.73 | 7.59 |
| Rh | 8.45 | 5.49 | 6.73 | 5.10 | 9.04 | 4.55 | 6.56 |
| Ru | 8.45 | 5.49 | 6.73 | 6.94 | 4.79 | 7.50 | 6.65 |
| Sc | 4.11 | 10.00 | 9.49 | 10.00 | 4.93 | 9.77 | 8.05 |
| Si | 0.00 | 5.37 | 6.36 | 2.04 | 5.21 | 10.00 | 4.83 |
| Ta | 5.15 | 2.89 | 3.57 | 2.04 | 5.34 | 9.77 | 4.79 |
| W | 5.35 | 7.24 | 8.58 | 3.67 | 10.00 | 0.45 | 5.88 |
| V | 3.37 | 4.43 | 5.15 | 3.27 | 5.07 | 0.00 | 3.55 |
| Y (HREEs**) | 3.93 | 10.00 | 9.49 | 10.00 | 4.93 | 9.77 | 8.02 |
Since CRMs may be contained, in different amounts, in the material composition (say, metallic alloy), the material criticality index can be defined as follows:
where n is the number of CRMs in the material chemical composition and wt%CRMi is the amount of the CRM ‘i’ measured in weight percent. It is noted that the criticality index (CI) represents an overall criticality value per unit of mass of the material.
Materials selection in a CRMs perspective
Once the overall material criticality is assessed (Eq. 5), the objective equation for the material index calculation in the frame of Ashby’s method is:
Since CI defines the criticality per unit of mass of the material (i.e.: a generic alloy), m* quantifies the criticality of the whole component in a CRMs perspective. By using the example described in the introduction, it is easy now to demonstrate that the material index for a rigid and low-criticality tie rod is:
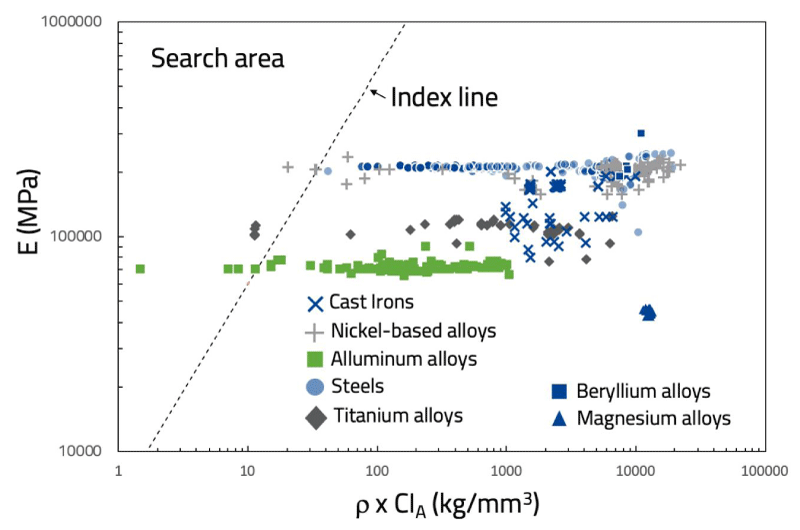
In the so called Ashby’s maps, that are log-log plots showing the position of different materials in the space defined by two materials properties (or combination of them) (Figure 2), Eq. (7) is a series of parallel straight lines of slope 1 (index lines). As M value increases, the index line moves toward the top left corner of the map. Materials on the left of the index line (search area) are of interest. By increasing the M value the search area narrows and selects the materials that optimize the objective [4]. This approach can be easily extended to design for recycling [5], material substitution [3] as well as design for environment in a CRMs perspective [6].
Figure 2: Metallic materials map for material selection in a CRMs perspective.
The 21st century challenges related to a new economy that respects the environment and resources can be tackled by an excellent knowledge of materials and even the acquisition of new skills that allow engineers and designers to apply mitigating actions against resource and energy consumption. In this scenario, a systematic strategy to select materials in a critical raw materials perspective was developed. The proposed strategy is based on the material criticality index definition that in turn allows defining an objective equation for the material index calculation following the Ashby’s procedure. The method is particularly suitable for the application of mitigating actions against CRMs intensive use (recycling, substitution, material efficiency).
This work is part of the results of the European project called ‘Design of Components in a Critical Raw Materials Perspective’ (DERMAP, KAVA project # 17205). Authors want to thank EIT Raw Materials for the financial support and all the Project Partners (SWEREA SWEECAST AB, Mondragon University, AGH University, EURECAT, Enginsoft, Fonderie Zanardi) for their contribute to the project development./p>
- Ashby MF, Bréchet YJM, Cebon D, Salvo L. Selection strategies for materials and processes. Materials & Design. 2004; 25: 51-67.
- Ferro P, Bonollo F, Cruz SA. Alloy Substitution in a Critical Raw Materials Perspective. Frattura ed Integrità Strutturale. 2020; 51: 81-91.
- Study on the review of the list of Critical Raw Materials. Critical Raw Materials Factsheets. 2017; ISBN 978-92-79-72119-9.
- Ferro P, Bonollo F. Materials selection in a critical raw materials perspective. Materials and Design. 2019; 177: 107848
- Ferro P, Bonollo F. Design for Recycling in a Critical Raw Materials Perspective. Recycling. 2019; 4: 44.
- Ferro P, Bonollo F, Cruz SA. Product design from an environmental and critical raw materials perspective. Int J Sustainable Engineering. 2020.