A new theory on the shape of the universe and the origin of the time

In this article, we have made a theoretical research, at the limit of the scientific reflection, to respond to the following query: what is the origin of the time? To find the response to this question, we adopted an attractive method. First, we have considered the light physical characteristics (speed, energy...) as the invariants. On this basis, we characterized all other physical systems by referring to the light. By this scheme, we showed that the calculation of the absolute physical characteristics of any system comes compulsory by the light viewpoint. That method avoids the use of mathematical transformations like Lorentz transformation; which is used in relativity to make invariant the equations by the change of the system of reference. Second, we made other hypothesis to find a law characterizing the interactions between matter and antimatter. Third, we used these interactions to quantiF_y the time. The central query of this research led us to the shape of the universe and its volume. Finally, we found that the universe is a conical shape.

The evolution of the science, particularly the evolution of physics, has been made by the scientists who brought useful ideas completely different of the old concepts. For example, the geocentric system of Ptolemy has been replaced with the solar system of Galileo [1]. Many other examples illustrate that evidence. As proof, the battle of ideas is the fuel of the scientific progress.

Nowadays, the time and the antimatter [2] are the two concepts which unite and divide the researchers. Nevertheless, the time is one of the fundamental concepts in which are operated the physical phenomenons. The thermodynamic [3] and the Newton mechanics [4] both adhere to an absolute interpretation of the time. Though the relativity of Einstein [5] presents a relative formalism of the time, it doesn’t really explain its origin. So, it naturally appears for physicists that the time renews perpetually; its motor turns continuously without stopping. That idea is sustained by the law of causality which forbids the time travels; clearly, it forbids the possibility to return to a passed instant [6]. At this step, we see that the time has a particular physical meaning. So, what phenomenons operate behind the time?

In this article, we will respond to the query related to the origin of the time and its nature. For that, we will determine the invariants of the universe by characterizing light (its speed, its energy...). AF_Terwards, we will characterize all other physical systems by referring to these invariants. Then, we will associate to these constants a single law which describes the interactions between the matter and the antimatter. Finally, we will also talk about a new particle we discovered on the way of this research query. AF_Ter all that, the last step of this paper will be focused on the shape of the universe.

Absolute speed

According to the Newton’s mechanics, the space and the time are two different separated concepts [7]. That means that the physical phenomenons evolve in an absolute time with the same pace everywhere in the universe. In 1905, Albert Einstein published an article on the special relativity theory. He explained that the space and the time are both linked and could not be studied separately. Thus, he defined a new concept called the space-time [8]. However, since Galileo the time and the space are connected by the speed. In this article, we use the variable t/x to designate the space-time. The variable t refers to the time and the variable x refers to the space.

In reality, the special or the general relativity is the theory of the absolute. Einstein has introduced the Lorentz transformation to make invariant the equations (Mechanics and Electromagnetism) by the change of the system of reference. In this paper, we do the same thing differently. We will establish absolute equations. For that, we adopt the fact that the speed of the light is invariant; it does not change whatever the observer. By the speed of the light, we evaluate the speed of the other physical systems. That is the way to find the absolute speed. In fact, the absolute values are dictated by the light. We also indicate that in this article we use the following hypothesis: the photon (particle of light) has a mass [9] (we will calculate its value later).

Now, we consider in the space-time a physical system (M) of mass m in movement. Its absolute speed is uniquely given by an immobile observer on the ray of light. We consider u that speed, m_ph the mass of the photon and c the speed of the light. The absolute speed of the system (M) is given by the equation (1). That means more a system is heavy more its speed is small. k_m represents the factor of mass.

$u= \frac{1}{k_m}c \left[m.s^{-1}\right] with k_{m }= \frac{m}{m_{ph}}$ (1)

Intern force

The space-time of the general relativity theory is smooth and continuous. It deforms with the presence of the matter [10]. More a physical system is heavy more the deformation of the space-time is great. By analogy with the intern energy, we call intern force the force of the mass. The measure of the intern force is proportional with the deformation of the space-time t/x. That evidence leads to conclude that the force is simply an integral (deformation) of the space-time. By integrating the variable t/x, we find the force (2).

$F_{m }= \frac{A}{2u^2} \left[N\right] with u= \raisebox{1ex}{$x$}\!\left/ \!\raisebox{-1ex}{$t$}\right.$ (2)

The photon is a particle with mass. It also creates a deformation of the space-time. Its intern force F_ph is calculated by substituting u to c in the equation (2). The expression of F_ph is given by the equation (3).

$F_{ph}= \frac{A}{2c^2} \left[N\right]$ (3)

Intern energy and temperature

By analogy with the precedent result, the intern energy is an integral of the intern force. We consider that the intern energy of a physical system E_m is conserved as the heat and is realized as the work.

$E_{m }= \frac{A}{6u^3} \left[J\right]$ (4)

The intern energy of the photon E_ph is given by the equation (5).

$E_{ph }= \frac{A}{6c^3} \left[J\right]$ (5)

By the same approach, we find the expression of the temperature T_m. For that, we use the Fourier’s law. The temperature is an integral of the energy (6).

$T_{m }= \frac{A}{24u^4} \left[K\right]$ (6)

Invariants of the universe: photon

We already know that the speed of the light is constant and absolute. By this affirmation, we adopt in this article another hypothesis: All physical quantities which characterize the state of the photon in its movement (speed, mass, momentum, force, energy, temperature) are constant and absolute. Thus, we will evaluate the physical characteristics of other systems by referring to the characteristics of the light.

In reality, the energy of photon is effectively constant. The wavelength λ, badly interpreted as the sign of the wave, is in reality the distance between two photons. That means that light is not a wave but a complex of particles called photons.

Now, let see how we can adopt the energy of photon without considering light like a wave. If the photons are near one to another (λ is small), their energies sum. Consequently, the electromagnetic radiation presents an intense energy. That is the case of the ultraviolet. If the photons are far one to another (λ is great), the radiation has a weak energy. So, we conclude that the energy of the electromagnetic radiation E varies proportionally with the energy of photon E_ph; and also varies inversely proportional with the wavelength λ (distance between photons) like shown in the equation (7).

$E= \frac{E_{ph}}{\lambda } \left[J\right]$ (7)

$E=h\frac{c}{\lambda } \left[J\right]$ (8)

By equaling the Yandza’s equation (7) with the Max-Planck’s equation (8), we determine the energy of photon E_ph (9).

$E_{ph}=hc \left[J\right]$ (9)

By another view, the photon is a particle with the lowest energy in the universe. We admit that the value of its energy E_ph corresponds to λ = 1 meter in the Max-Planck’s equation (8). That means that the wavelength of any radiation is always small than one meter. So, photons cannot interact each other beyond that distance.

$E_{ph}=m_{ph}{c}^2 \left[J\right]$ (10)

By equaling (9) and (10), we determine the mass of the photon m_ph like given by the equation (11).

$m_{ph}= \frac{h}{c} \left[Kg\right]$ (11)

The momentum of the photon p_ph is also invariant. Its value is given by the equation (12).

$p_{ph}=h \left[Kg.m.s^{-1}\right]$ (12)

By equaling (5) and (10) we determine the value of the integral constant A like shown by the equation (13).

$A=6h{c}^4 \left[J.m^3.s^{-3}\right]$ (13)

The equations (2), (4) and (6) become respectively (14), (16) and (17). The equation (15) is deducted of (3). The equation (18) is deducted of (6).

$F_m= k_m^2{F}_{ph } \left[N\right]$ (14)

$F_{ph }=3h{c}^2 \left[N\right]$ (15)

$E_m= k_m^3{E}_{ph } \left[J\right]$ (16)

$T_m= k_m^4{T}_{ph } \left[K\right]$ (17)

$T_{ph}= \frac{h}{4} \left[K\right]$ (18)

The Table 1 presents the physical quantities that we call the invariants of the universe. The volume of photon V_ph is calculated in section 3.

Yandza’s law

To introduce the Yandza’s law, we first mention what Newton states. Newton declares that any physical system in movement, under the action of an exterior force F_ext, is attracted by the earth. The force of this attraction is proportional to the mass of the physical system [11] like shown by the equation (19).

${{\displaystyle \Sigma }}^{\text{}}{F}_{ext}=m \gamma$ (19)

Now, we consider a physical system (M) of mass m in movement. According to Newton, (M) is under controlled by the force of the earth F_T [11]. In section 2, we defined the intern force as the force of mass, responsible of the movement without any other exterior force. By the way of this definition, we see that the force with it the earth exerts the attraction on the moon and the other systems is simply its intern force (if we neglect the influence of the sun).

Now, let see clearly the interactions between the earth and the system (M) of mass m, intern force F_m. We consider that (M) levitates like a satellite. By studying the linear movement of the system (M) in the space, we observe that everywhere the planet goes the system (M) is. It becomes clear that (M) is linked with the earth. So, we also consider that the liaison between them is rigid. So, the evidence shows that the absolute speed of the earth is equal to the absolute speed of the system (M). According to the equation (2), the equality of the speeds leads to the equality of the intern forces (F_m = F_T). In another view, the equation (1) specifies that the earth and the system (M) could not have the same absolute speed because their masses are different. The only case in which the system (M) reaches the speed of the earth is related to the appearance of an acceleration in its movement. With this acceleration is engaged the acceleration force F_γ. So, the balance of forces applied to the system (M) is shown by the equation (20).

${{\displaystyle \Sigma }}^{\text{}}{F}_{ext}+ F_m+ F_{\gamma }= F_T$ (20)

The equation (20) is incorrect; because only the intern force of the earth F_T is considered as the cause of the attraction. In reality, the source of the attraction comes also by the interactions with the other systems. First, the earth is attracted by the sun and develops an acceleration to reach the intern force of the sun F_s (like was doing the system (M)). Second, the sun is accelerated by the galaxy (the Milky Way). That implicates a new acceleration. Third, the Milky Way is also accelerated by the rest of the galaxies of the universe. That will also increase the acceleration of the earth. This analysis can be applied to any other physical systems in the universe. The new balance of forces applied to the system (M) is shown by the equation (21).

${{\displaystyle \Sigma }}^{\text{}}{F}_{ext}+ F_m+ F_{\gamma }= F_T+F_{\gamma s}+\dots + F_{\gamma \infty }$ (21)

F_γs: acceleration force of the earth under the influence of the sun

F_γ∞: acceleration force of the earth under the influence of the infinity

By the equation (21), we can see that any physical system in the universe develops an acceleration (except the light) under the influence of only one and same invariant force. This is the statement of the Yandza’s law.

That invariant force is the one we know: the intern force of the photon F_ph. It represents the density force of the universe. The light does not accelerate because it has already reached the force F_ph. The intensity of F_ph is very small than the intern forces of the planets and the stars in the universe. For justifying the equation (21), we introduce a negative force F_ap (force of antiparticles) in the balance of forces. The result is presented by the equation (22).

${{\displaystyle \Sigma }}^{\text{}}{F}_{ext}+ F_m+ F_{\gamma }-F_{ap}= F_{ph}$ (22)

By (22) we find that the space-time produces the antiparticles of the mass equal to the mass of the photon (the smallest in the universe). We call these antiparticles: antiphotons.

The equation (22) is not instantaneous. It operates progressively. That means that the equality is verified at the end of the phenomenon. Due to the interactions between matter and antimatter, the equation (22) reveals the disintegration of the initial mass to the state of the photon [12]. However, at each instant of the disintegration, an equality is verified in term of mass, momentum, force and energy. The equations (23), (24), (25) and (26) present these equalities.

The mass associated to the acceleration M_γ has no matter. That term is added in the equation (23) to justify the variation of the absolute speed. That means also by accelerating a physical system, its mass changes. The value of M_γ is found by measuring the difference of mass ∆m between two instants. To this variation of mass corresponds the speed related to M_γ like shown by equation (1). By the same approach, we deduct p_γ, F_γ and E_γ.

$\Sigma m+ M_{\gamma }- M_{ap}^r- M_{ap}^p= k_m{m}_{ph}$ (23)

$\sum p_{ext}+ p_{m,i}+ p_{\gamma }- p_{ap}^r- p_{ap}^p= k_m{p}_{ph}$ (24)

$\sum F_{ext}+ F_{m,i}+ F_{\gamma }- F_{ap}^r- F_{ap}^p= k_m^2{F}_{ph}$ (25)

$\sum E_{ext}+ E_{m,i}+ E_{\gamma }- E_{ap}^r- E_{ap}^p= k_m^3{E}_{ph}$ (26)

Particle Y

Now, we consider that the precedent system (M) is made of antimatter. All the remarks we made stay applicable. But, instead of generating a flux of antiphotons (negative force $F_{ap}^p)$ ), the system (M) will produce a flux of photons (positive force $F_{ap}^p)$ ). So, in one case (matter) we have the production of antiphotons and in another one (antimatter) we have the production of photons [13]. In the vision to unit these two kinds of production, we postulate that only one particle is emitting. We call that particle: particle Y.

The particle Y has the property to transform into an antiphoton when matter is around; and to transform also into a photon when antimatter is surrounded it. Its mass is equal to the mass of photon or antiphoton m_ph. By opposition to the photon, the antiphoton has a negative energy.

In general, the equations (23), (24), (25) and (26) become (27), (28), (29) and (30). When the initial mass is made of antimatter, the following terms are negative:m, M_γ, p_m,i, F_m,i, and E_m,i. The sign of the terms associated to the exterior solicitations depends on the origin of the solicitation (matter or antimatter). We also speciF_y that if the system produces only the antiphotons, that means $M_{ph}^p=0$ . We apply the same rule when the system produces only photons,$M_{ap}^p=\text{0}$ . If the studied system is made of matter and antimatter, the two kinds of productions operate simultaneously. If the system does not receive any matter and antimatter, the quantities $M_{ph}^r$ and $M_{ap}^r$ are equal to zero.

$|\Sigma m+ M_{\gamma }+ M_{ph}^r+ M_{ph}^p- M_{ap}^r- M_{ap}^p|= k_m{m}_{ph}$ (27)

$|\sum p_{ext}+ p_{m,i}+ p_{\gamma }+ p_{ph}^r+ p_{ph}^p - p_{ap}^r- p_{ap}^p |= k_m{p}_{ph}$ (28)

$|{{\displaystyle \Sigma }}^{\text{}}{F}_{ext}+ F_{m,i}+ F_{\gamma }+ F_{ph}^r+ F_{ph}^p - F_{ap}^r- F_{ap}^p|= k_m^2{F}_{ph}$ (29)

$|\sum E_{ext}+ E_{m,i}+ E_{\gamma }+E_{ph}^r+ E_{ph}^p - E_{ap}^r- E_{ap}^p |= k_m^3{E}_{ph}$ (30)

Time

According to the results we found in this section, the space-time produces matter and antimatter by emitting the particles Y. However, the equations (27), (28), (29) and (30) show that at the appearance of a particle (or an antiparticle) is engaged accounting of time. In fact, without the initial mass, there is no production of photons or antiphotons. Without these productions, there is no disintegration of the initial mass to the state of a photon. So, the time is directly linked to the production of particles Y.

For a system made of matter, the time is the measure of the interaction between matter and the total mass of the antiphotons produced. For a system made of antimatter, the time is the measure of the interaction between the antimatter and the total mass of the photons produced.

For measuring the time t needed to disintegrate a matter to the state of a photon, it is compulsory to determine the factor of mass k_Y associated to the production of photons and antiphotons; because at the emission of one single photon (or antiphoton) is corresponding one unit of time like shown by equations (31) and (32). We insist on the fact that the mass of the antiphotons (or photons) received does not enter in the calculus of the time; because its effect on the physical system is instantaneous. That effect is already considered in the mass balance (27).

$t= k_Y= \frac{M_{ap}^p}{m_{ph}} \left[s\right]$ (31)

$t= k_Y= \frac{M_{ph}^p}{m_{ph}} \left[s\right]$ (32)

Shape of the universe

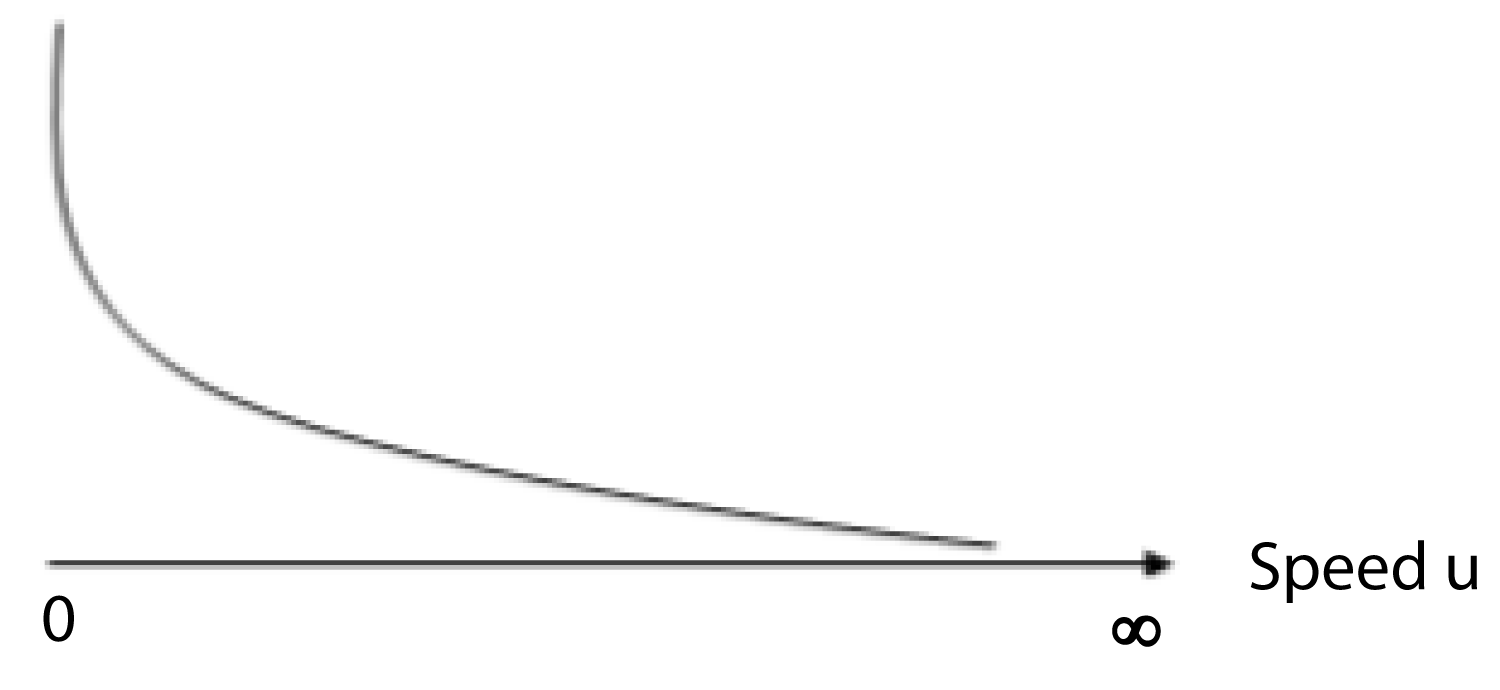
The equation (2) presents the relation between the intern force of a physical system and its absolute speed. According to the Yandza’s law, any system in the universe develops an acceleration under the influence of only one and same invariant force. That means that the speed of any system is not constant. Thus, we can draw the curve of the intern force (Figure 1).

We have considered the force as the deformation of the space-time. By summing all these lines of force in the universe and by applying the symmetry, we discover that the universe is a conical system (Figure 2).

Volume of the universe and photon

The volume of the universe V_u is obtained by integrating the shape of the universe like presenting by the Figure 2. The integral calculus is presented by the equation (33) and the final result by (34). F_u is the intern force of the universe and k_u is its factor of mass.

$V_u= {\int }_0^{Fu}rdr {\int }_0^{2\pi }d\theta {\int }_0^{c}du$ (33)

$V_u=9\pi h^2{c}^5{k}_u^4 [m^3]$ (34)

Similarly, with the equations (14), (16) and (17), we deduct the volume of the photon V_ph (or antiphoton) (35). V_ph belongs to the invariants of the universe.

$V_{ph}=9\pi h^2{c}^5 \left[m^3\right]$ (35)

So, we can relieve the volume of a photon (or antiphoton) to the volume of the entire universe by the factor of mass (36).

$V_u= k_u^4 V_{ph} \left[m^3\right]$ (36)

In one unit of time, or one second, the distance that the photon can cover is equal to c meters. That evidence is found by the following equality: $c= \frac{c meter}{1 second}.$ So, the conical volume that the photon can colonize in one second V_max is given by the equation (37). The dimension of the related volume is shown by the Figure 5.

V_max = $\frac{\surd 3}{24} \pi c^3$ (37)

The proportion $\frac{V_{max}}{V_{ph}}$ represents the number of units obtained by filling the volume V_max by the volume of photon $V_{ph}$. That value is the maximal unit of volume. By the same rule, it comes evident that the proportion $\frac{V_{max}}{V_{ph}}$ is also the number of the volume V_max that can contain the volume of the universe V_u. So, the volume of the universe V_u is calculated by the equation (38).

$V_u= \frac{V_{max}}{V_{ph}} V_{max}$ (38)

By replacing V_max and V_ph by their quantity, we find the exact volume of the universe V_u (39). By the same approach we deduct the mass of the universe (40).

$V_u= \frac{\pi c}{1728 h^2}$ (39)

$M_u= \frac{1}{15552 h^3 c^{5 }}$ (40)

We find the volume of the universe V_u = 1,24140890.10⁷² m³ V_u and the mass of the universe M_u = 9,12729870.10⁷² K_g. These values are admitted for an isolated universe. Nowadays, physicists discovered the expansion of the universe. That does not make our values fail. By the equation (36), we know that the volume of the universe V_u can change only if the mass of the universe changes. That implies that the expansion of the universe means that another system outside of the universe brings mass to our universe. In other words, the expansion of the universe is the proof of parallel universes.

The very recent experiments revealed the mass of the photon as 10⁻⁵⁴ Kg [13]. That experimental value is not yet confirmed. That value has been specified when photon interacts with matter only. There is not a rich bibliography on this subject. However, the value we obtained 2,21021890.10⁻⁴² Kg shows that at the level of Max-Planck constant (10⁻³⁴ m), the mass of photon is clearly equal to zero. But in the deep scale, photon is not a massless particle. Its mass is exactly what we find in this research. We have also proved that photon and antiphoton do not accelerate because their intern force is invariant in the universe; like demonstrated in relativity [14].

The question related to the shape of the universe is central is this research. In our bibliography, we found two types of theories which develop a certain idea on the shape of the universe. The first one presents the universe like a flat space [15]. The second one attests that the universe is a spherical shape [16]. For now, we do not totally reject the conclusions of these researches. But, we think that in these two cases, the authors did not take account the effects of the intern forces applied in the universe. A flat universe or a spherical one turns to conical shape when intern forces are applied. That remark is a physical evidence on the basis of relativity theory [17] and the interpretation of the force in this paper. To make it clear, let consider a flat shape suspending in the air. When the ball falls on it, the shape deforms. If the pressure of the ball is continuously maintained on this shape, its global shape becomes conical. That is the same if we consider a spherical one. Like we see that any particle in the universe concurs to an acceleration to obey to Yandza’s law, so the shape of the universe is conical by the fact of the intern forces.

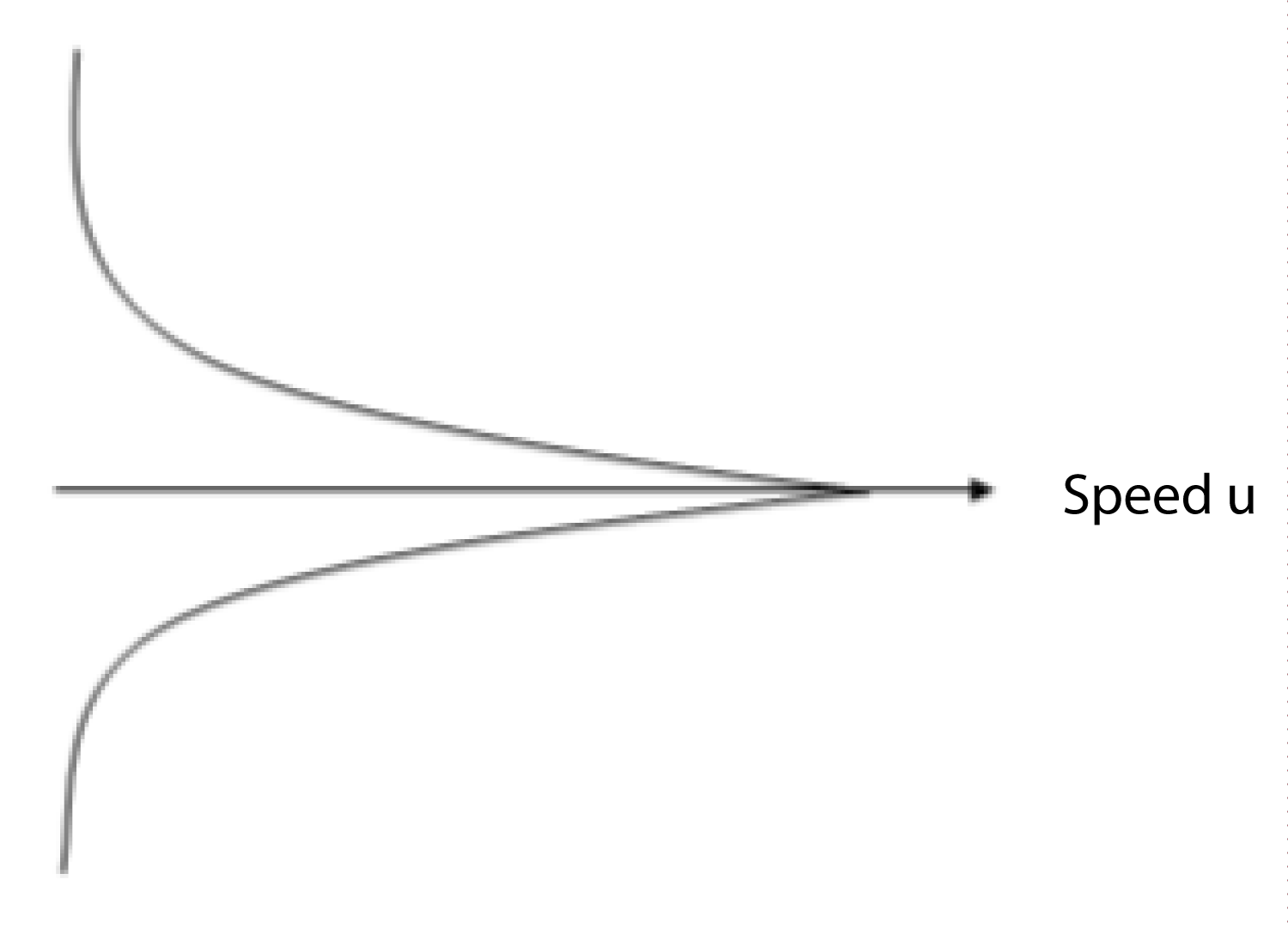
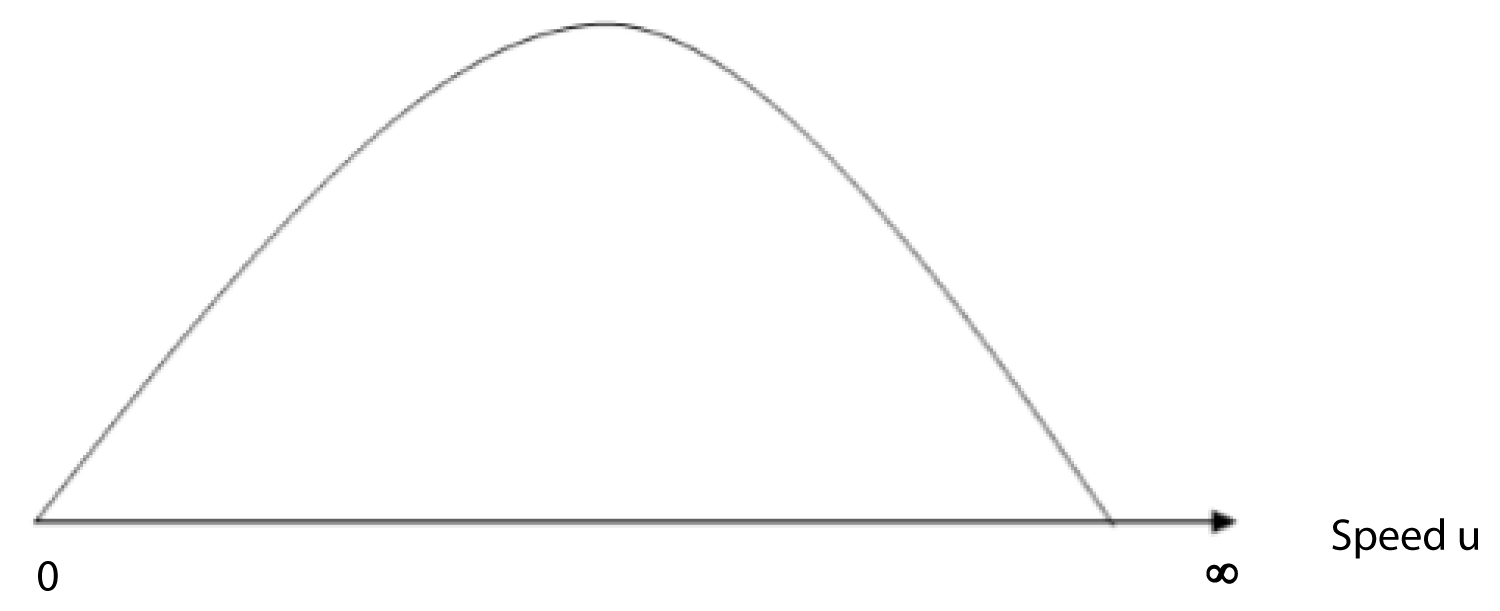
To conclude this precedent paragraph, we completely rejected that the universe is a spherical or a flat shape. First, a spherical universe obliges the intern force having the representation of the Figure 3. It is not a correct shape because the intern force presented in this article continuously reduces when the speed increases with the acceleration. But in the case of spherical universe, the intern force increases and then decreases after attaining its extremum. That would state that big stars like the sun (with extreme low speed) have approximately the same intern force than the photon (with extreme high speed). That is completely false. Second, in the same line, by considering a flat universe (Figure 4), no acceleration can be operated. The intern force is always constant. That is opposed to Yandza’s law. It would mean that the intern force of the sun for example is continuously equal to the intern force of the photon. On the basis of these arguments we effectively conclude that the universe has a conical shape.

In addition, many authors state that the universe is an infinite system [18]. This idea is opposed to the big bang theory because the infinity cannot have an origin. According to the equation (36), an infinite universe is possible only if k_u stretches to infinity (m_ph = 0). We disagree with that option and adhere to the second community of scientists who think that the universe is finite [19]. The finite universe is in accordance with the big bang theory and recognized that photon has a mass like we developed in this paper.

In this article, we presented the invariants of the universe. There are all the characteristics of the light: its speed, its mass, its volume, its momentum, its intern force, its intern energy and its temperature. The balance of any system in the universe depends on these invariants. We use these constants to characterize the other physical systems. By this approach we found the absolute values.

We have also shown that any physical system in the universe develops an acceleration under the influence of only one and same invariant force. We called that principle the Yandza’s law. On the basis of that law, we defined the time as the measure of the interactions between the matter and the antimatter. By studying these interactions, we discovered a new particle which disintegrates to a photon or an anti-photon according to its environment. We called that particle: particle Y.

To conclude this research, we defined the force as the measure of the deformation of the space-time. So, we drawn the lines of force and we discovered the conical shape of the universe.

1. Galilei G. The Essential Galileo, Hackett Publishing Company, 2008.
2. Benoit-Levy A. Etude de la concordance d’un univers de Dirac-Milne symétrique matière-antimatière, Thèse de doctorat, université Paris-sud. 2009.
3. Perez JP, Thermodynamique: Fondements et applications, Masson, Paris. 1997 ; 96-116.
4. Schroeder O. La loi de la gravitation universelle Newton Euler et Laplace, Springer, 2007.
5. Perez JP. Relativité: Fondements et applications, Dunod, Paris, 2016.
6. Frank P. The law of causality and its limits, Springer Science + Business Media Dordrecht, 1998.
7. Haug EG. Newton’s and Einstein’s gravity in a new perspective for Planck masses and smaller sized objects. Int J Astron Astrophys. 2018; 8: 6-23.
8. Motohashi H, Minamitsuji M. General relativity solutions in modified gravity. Phys Lett. 2018; B781: 728-734.
9. Tu LC, Luo J, Gilles GT. The mass of the photon. Reports on Progress in Physics. 2004.
10. Arruga D, Achour JB. Deformed general relativity and quantum black holes interior, Universe, 2020.
11. Newton I. Mathematical Principles of Natural Philosophy, published by Daniel Adee, 1846.
12. Abdel-Raouf M. Novel consequences of coexistence of matter and antimatter in nature. J High Energy Phys Gravitat Cosmol. 2020; 06.
13. Coray M, Annavarapu R. Rest mass of photon on the surface of matter. Results Phys. 2020; 16: 102866.
14. Hartle JB. General relativity in the undergraduate physics curriculum. Am J Phys. 2006.
15. Kanjampatti PO, Nadu PT. On freedman equation and the shape of our universe. Ann Mathemat Phys. 2020.
16. Luminet JP. The shape and topology of the universe. arXiv, 2008.
17. Hojman S, Kuchar K, Teitelboim C. New approach to general relativity. Nat Phys. 1973; 245: 97-98.
18. Borchardt G. Infinite universe theory, Proceedings of the NPA. 2015.
19. Luminet JP. Weeks JR, Uzan JP. Dodecahedral space topology as an explanation for weak wide-angle temperature correlations in the cosmic microwave background. Nat. 2003; 425: 593-595. PubMed: https://pubmed.ncbi.nlm.nih.gov/14534579/